Creep Crack Growth Properties of P91 Parent and Welded Steel

TWI Ltd
Granta Park, Great Abington
Cambridge CB21 6AL
UK

Kamran Nikbin
Imperial College London
South Kensington Campus
London SW7 2AZ
UK

Paper presented at WELDS 2009: Design, testing, assessement and safety of high-temperature welded structures. Fort Myers, FL. USA. 24-26 June 2009.

Abstract

Old grades of creep resistant materials such as P11 and P22 have been studied in depth and data and prediction models are available for design and fitness for service assessment of creep rupture, creep crack growth, thermo-mechanical fatigue, etc. However, as the 9%Cr material is relatively new, there is relatively limited data available and understanding with respect to quantifying the effect of variables on life prediction of components fabricated from P91 is more difficult. Since Grade P91 steel was introduced in the 1980s as enhanced ferritic steel, it has been used extensively in high temperature headers and steam piping systems in power generating plant. However, evidence from premature weld failures in P91 steel suggests that design standards and guidelines may be non-conservative for P91 welded pressure vessels and piping. Incidences of cracking in P91 welds have been reported in times significantly less than 100,000 hours leading to safety and reliability concerns worldwide. This paper provides a review and reanalysis of published information using properties quoted in codes of practice and from recent research data regarding the creep crack growth of P91 steel, and uses existing models to predict its behaviour. Particular areas where existing data are limited in the literature are highlighted. Creep crack growth life is predicted based on short-term uniaxial creep crack growth (CCG) data. Design and assessment challenges that remain in treating P91 weld failures are then addressed in light of the analysis.

Keywords: P91; Creep crack growth; fracture mechanics, NSW; NSW-MOD; C*

Introduction

Modified 9Cr-1Mo alloy, known as Grade P91 steel, has been extensively used for high pressure and high temperature piping and headers in conventional power plants mainly in the outlet section of the boiler such as final superheater and also main steam piping which are subject to the creep damage.^[1] The weld P91 material, which is also subject to damage could be the weakest link in the structure and would need to have validated laboratory data available in order to make meaningful life assessment predictions.

Although creep life of most components is characterised by a continuum damage mechanism CDM where failure is controlled by either creep rupture or creep strain failure mode, creep crack initiation (CCI) and growth (CCG) in some cases tend to dominate the total life of the component.^[2] Examples of this situation include components containing fabricating flaws or thick components such as headers, high pressure/temperature piping and body of the high temperature hydroprocessing reactors.

In both power generation plants and the chemical industries there is, therefore, a need to assess the significance of defects which may exist in high temperature equipment operating in the creep regime. With further understanding of creep crack growth behaviour and improvements in NDE methods, fracture mechanics assessment approach is increasingly being used. This method assumes the presence of a crack of finite size in a component and then evaluates its propagation due to creep to determine the remaining life of the component. This approach also is widely used for fitness for service assessment of components known to contain crack like flaws. Several design and assessment procedures are available for CCG assessment such as British Energy R5, ASME/API RP 579, BS 7910, French RCC-MR (Appendix 16) etc.^[3-6]

Predictive models for steady-state creep crack growth

At elevated temperature metals exhibits a stress dependent deformation rate. This high temperature deformation (creep) rate may be related to the stress by a power law;

ė = Aσⁿ  

[1]

This deformation is usually composed of three regions, known as primary, secondary (or steady-state) and tertiary creep stage. In practical applications, service load and temperature, the steady-state region usually dominates the life of the component subjected to creep deformation. For a cracked body operates in elevated temperature where creep is dominant, time dependant crack growth is observed. To identify the CCG behaviour in such component, several fracture mechanics parameters have been applied such as stress intensity factor, K, and creep fracture mechanics parameter C* integral. The relationship for growth rate given for steady-state creep dominant conditions in engineering alloys has been shown to be ^[7]:

The selection of a suitable parameter to describe crack growth at elevated temperature will depend on material properties, loading condition, size, geometry and the period of time during which crack growth is observed.^[8]

For a power law creeping material which follows equation (1), the steady crack tip stress field and strain rate distributions at coordinates (r,θ) are expressed by ^[7]:

By assuming a creep process zone at a crack vicinity, the material starts to experience creep damage when it enters the process zone at r = r_c , at the time t=0, and accumulates creep strain ε_ij ^c by the time it reaches a distance r from the crack tip, the condition for crack growth is given using the ductility exhaustion criterion as;

If it is implicitly assumed that failure occurs at the crack tip when the available material creep ductility is exhausted at the value of Image.1. at which Image.2. reaches its maximum value of unity ?_ij = 1), integrating for a constant growth rate at constant C* gives ^[7];

 

Image.1.

Image.2.

This model is known as NSW model and indicates that crack growth rate should be inversely proportional to the creep ductility ε^* _f appropriate to the state of stress at the crack tip. To estimate the ratio of the multiaxial to uniaxial failure strain, ε^* _f / ε_f, using Cocks and Ashby void growth and coalescence model suggests for most relevant engineering materials, the ratio between the extreme multiaxial, plain strain, and uniaxial, plain stress conditions is recommended to be a factor of 30.^[9] This assumption is used in the analysis work carried out in this paper.

Nikbin et al^[10] demonstrated that the power dependence of C* varies only over the range 0.7 to 1.0 and that crack growth rate can be predicted for plane stress conditions approximately within a factor of about 2 by:

Equation (8) is called NSW engineering creep crack growth law where the predicted bounds cover the extreme conditions of stress state and creep ductility.

In the NSW model the value of the non-dimensional equivalent stress function, in equation (4), Image.3. was taken to be its maximum value of unity. This is considered to be a very conservative measure and implicitly assumes that failure will occur first at the angle, Image.4. attains its maximum value. Figure 1 ^[11] shows the dependence of crack-tip stress field and ε_f / ε^* _f on θ under plane stress and strain. It is seen from Figure 1 that the maximum value of Image.5. is unity at θ ≈ 0° and 90° under plane stress and plane strain conditions, respectively. At θ = 0°, which is the condition assumed in the NSW model, the difference in the value of Image.5. under plane stress and plane strain conditions can be up to a factor of 50-100 depending on the value of n. It is also seen in figure 1(b) that ε^* _f increases with angle both under plane stress and plane strain conditions. At θ = 0° the difference of ε^* _f between plane stress and plane strain conditions is well above a factor of 100.

Image.3.

Image.4.

Image.5.

Fig.1. Dependence of
a) 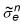   and
b) ε^* _f on angle θ and n

This variation will also affect the value of ε^* _f which it will vary for a range of stress states. A more general expression can be obtained, which considers the dependence on angle, θ and failure is considered to occur first where the ratio of angular function of the equivalent stress (Image.6.) and the multiaxiality strain factor (ε_f / ε^* _f) attains its maximum value. For this condition, the NSW model may be extended to give a modified crack growth rate, referred to as the 'NSW-MOD model ^[11]:

Image.6.

Comparison of the CCG rate under plain stress and plain strain conditions for creep ductile material (n>5) shows the maximum value of CCG rate under plane strain conditions is about 3 to 7 times greater than that under plane stress, although the ratio depends on the value of n.

A material independent engineering creep crack growth (CCG) assessment diagram (see Figure 2) normalised against failure strain highlights the range of cracking that can be predicted in a wide range or metals.^[12] Figure 2 illustrates that the scatter band of CCG data falls between an upper band controlled by plane strain condition and lower band controlled by plane stress condition. There are number of factors affecting this estimation such as the formula adopted for assessment ^[13], test condition ^[14], laboratory specimen geometry and size creep ductility. The difference between plane stress and plane strain CCG based on NSW model for the creep ductile material was obtained to be a factor of 30. However this is reduced to a factor of approximately 6-7 for n values of 7-10 when using NSW-MOD model as shown in a lighter shade data band in Figure 2.

Fig.2. Material independent creep crack growth engineering assessment diagram

Sensitivity of creep crack growth

Prior to the discussion regarding the effect of different variables on CCG rate, it is necessary to examine the effect of CCG data variation on the assessment life for components operating at high temperature. To illustrate the sensitivity of the assessment results to the CCG data, two extreme values of NSW model, which are the plane stress and plane strain conditions as the lower and upper band data, are used. The example from BS7910^[5] Appendix U was used to present this effect using P91 material data. The example was simplified by assuming the steady-state loading condition. Table 1 summarises the relevant parameters for P91 material and the input data used in the calculation and Figure 3 illustrates the results from the assessment.

Table 1 Information used in the P91 example

 

Information used in the P91 example
Thickness	35mm
Temperature	550°C
Flaw type	Surface breaking crack
Crack length (c)	20mm
Crack depth (a)	7mm
Primary membrane stress	90MPa
Primary bending stress	0
Secondary stress	0
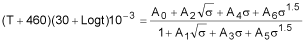
Creep rupture data [4]	In the above formula, the unit for σ is ksi, T is in degree Fahrenheit and t is in hours.
Plain stress CCG rate [5]	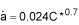Where C* is in unit N/(mm.h)
Plain strain CCG rate	Where C* is in unit N/(mm.h)

Fig.3. Example for sensitivity of life assessment to the data scatter band for a P91 component based on BS7910 procedure Appendix T

As the figure indicates, by assuming a factor of 30 on CCG rate for plain strain condition, the component is considered to be unsafe in five months time of operation, whereas using the plane stress data allows the operation to be continued beyond nine years time. This level of uncertainty could either have an enormous financial impact or a serious safety concern.

As mentioned in the previous section, similar to all mechanical testing, it is a common practice to use laboratory tests results to estimate the CCG behaviour of an actual in-service component. Therefore there is a need for validating CCG data produced under laboratory condition and comparing them to failures in component. The two major issues with laboratory testing are test condition and the characteristics of specimen which needs to represent the actual condition. Due to stress sensitivity in creep, any error in testing is magnified in the analysis. For example it has been demonstrated that 1% variation in load can lead to 3% to 10% variation in crack growth rate. However measurement of crack size and displacement rate has even more significant influence on the results. ^[14]

 

The choice of using different formulae to calculate reference stress (σ _ref ) and C* is another source of variations in CCG rates Variations in crack growth rate up to a factor of 2 have been found from different reference stress solution. Using the minimum or average material properties could vary the crack growth rate prediction by up to a factor of 5.^[13]

The CCG rate is controlled by creep ductility and constraint.^[7] In the case of laboratory testing, the size and geometry of the test specimen control the level of constraint. This paper reviews and analyses the published information and recent research data regarding the CCG of P91 steel. The analysis work highlights particular area where existing data are limited and design and assessment challenges remain.

Scatter band for P91 CCG data

As mentioned above, constraint and creep ductility are two major factors which control the crack propagation rate under the creep regime at a given service condition. Effectively, the geometry and inherent properties of the material determine creep ductility and level of constraint.

ASTM E1457^[8] provides different choice of specimen geometry for CCG testing. Extensive studies have been carried out to investigate the variation in creep crack propagation rate with specimen geometry and size. The most common specimen which has been used conventionally to measure CCG rate is Compact Tension (CT). Davis et al^[15] conducted CCG test on parent and welded 316H stainless steel using different size of CT specimen and the results were plotted against the creep fracture mechanics parameter, C^* . The result indicated no apparent size effect on CCG data when using CT specimen. In a similar investigation, Nikbin^[16] illustrated the same conclusion by plotting CCG data against C^* for P22 steel using CT specimen. Little influence of specimen size was observed and the data were collated within a reasonably narrow scatter band except for the early stage of the test which was attributed to the redistribution of the stress from elastic to a steady creep state at the crack tip. He also showed little influence of different temperature on CCG result. This was attributed to the fact that the CCG correlation depends primarily on creep failure strain.

It has been proven by a number of research works^[16-18] that, compared to feature component tests, a better correlation between CCG rates and C^* could be obtained when CT type specimen was used.. In a feature component test, the test is usually conducted on a component representing similar geometry and condition of a real component in service. However it has been claimed in^[16] that this correspondence is satisfactory except for shallow cracks in thin walled tube. It was also suggested that the lack of agreement may be attributed to an overestimate of C^* when using the reference stress procedure for shallow cracks, but further finite element analysis will be needed to provide confirmation. Wasmer et al^[16] used pre-cracked (external semi elliptical defects) in pressurised straight and bent pipe manufactured from P91 as feature component. Tests performed on straight and bent pipes showed the same behaviour and also little differences in CCG data was observed compared to the result from CT specimen.

In a similar investigation,^[18] CCG tests were performed on the internally pressurised seam welded pipe manufactured from P91 containing artificial axial crack-like flaws in different positions (Parent and HAZ). The CCG rate data was assessed in terms of both stress intensity factor and C^* . When using C^* , the assessment of CCG was found to be relatively independent of the stress state and the results provided better correlation with respect to conventional data generated using CT specimen. Most of the data generated in this experiment fall inside the Task 2^[19] scatter band. Unfortunately the report from Task 2 was not in a public domain; however the task 2 data provided in other reference [20] were used. CCG tests on P91 parent and HAZ were also performed within the large experimental program of the European Research Project, HIDA (high temperature defect assessment).^[20] Table 2 provides the CCG parameters extracted from these experiments for comparison. The CCG data for P91 parent material and HAZ from BS 7910 are also provided in Table 2 for comparison. The authors could not clarify the source of these data in BS 7910, however based on the wide literature review it is assumed that the data should be extracted by BS 7910 high temperature committee from the experimental results mentioned in this paper. Figure 4 illustrates the CCG rate data versus C^* from the above experiments together with BS7910 data for comparison. Plane stress and plane strain curves from NSW model in equation (7) are also presented to show the prediction band by the model.

Table 2 P91 CCG parameter from different references (CCG rate is in the unit of mm/h)

Reference	Material		Temp. °C	C* unit	εf	CCG rate parameters from Equation 4
						D	?
[18]	HAZ		625	MJ/m2h	-	-	0.803
[17]	Parent		600-700	MPa.m/h	0.07-0.32	1.44	0.6
	HAZ		600-625		0.31-0.42
	WM		600-625		0.03-0.07
	XW		600-675		0.015-0.17
[19]	Parent		-	MJ/m2h	0.13	1.8	0.63
	Weld		-		0.02	20	0.8
[20]	Parent		625	MJ/m2h	0.19	7.62	0.8
	Parent				0.21	4.39	0.68
	Parent				0.32	4.72	0.79
	Parent-HAZ				0.15	3.33	0.59
	Pipe bend				0.03	3.79	0.84
BS 7910 [5]	Parent	Upper band	580-593	N/(mm.h)	-	0.05	0.65
		Mean value				0.024	0.7
	HAZ	Upper band	580			0.091	0.78
		Mean value				0.046	0.78

Fig.4. Comparison of different P91 CCG data from literatures

Instant conclusion from Figure 4 is the majority of data are collected from plane stress condition. As Figure 4 indicates, the parent material data from^[5], ^[17], and^[19] provide the lowest level of CCG rate. Weld metal from^[19] indicates slightly higher CCG rate. The highest CCG rates belong to the data from BS 7910. ^[5]

 

The above data together provides an overall scatter band with an upper and lower limit. This could be identified as P91 current CCG scatter band. Figure 5 presents this overall diagram.

Fig.5. P91 data scatter band from all literatures, NSW model and NSW-MOD model

The P91 CCG parameters from equation (3) are provided in Table 3. These data belong to C^* values between 10^-6 MJ/m²h and 0.1 MJ/m²h.

Table 3 P91 overall CCG parameters from experiments

CCG parameter	Upper band	Mean	Lower band
D	17.22	6.44	2.3
?	0.72	0.72	0.804

It can be seen from Figure 5 that there is a wide variation in the P91 CCG data scatter band. The difference between upper band and lower band of experimental data in low values of C^* (10^-6 MJ/m²h) could be more than a factor of 20. This decreases to an order of magnitude in higher values of C* (0.1 MJ/m²h). The difference between upper band and mean value is a factor of 3 which is almost constant within the C^* values. The NSW prediction bound from equation (7) and NSW-MOD prediction bound from equation (9) are also shown in figure 5. The average value of n=10 is reported in^[20] for P91 material which allows using NSW-MOD model to predict its CCG behaviour (the value of n is reported to vary between 5 to 14 for the P91), however as it can be observed from the figure, although the there is a large scatter in P91 data scatter band from experiments, the prediction by NSW-MOD plain strain is still greater than the upper band P91 data particularly in greater value of C^* (C^*>10^-3 MJ/m²h) which implies the suitability of the predictive model as an upper bound limit. This scatter bound can be even greater value if the creep ductility of XWeld (0.03) is used. NSW model predicts greater scatter. The NSW plain strain predicts significantly greater value for CCG rate on all C^*. Both NSW and NSW-MOD plain stress predictions are reasonably within the data collected by experiments, however the predictions are higher in greater value of C^* (C^*>10^-3 MJ/m²h). This can be due to the duration of most experimental tests which are normally within few thousands hours and the results are extrapolated to longer term service exposed material.

The data presented in this review were collated from data in the range of 10^-6 <C^* (MJ/m²h) < 10^-1m. Equation (5) can be used to evaluate the value of strain rate local to the crack tip corresponding to C^*, taking a maximum value of unity for Image.1. at the failure time;

Substituting the value n=10 and A=10^-27 used in Figure 4 and 5 into the equation (10) for C^*=10^-6, provides the constraint of the data to the minimum strain rate equal to 5.6 x 10^-5 h^-1 for plain stress condition and 7.6 x 10^-5 h^-1 in plain strain condition. This relates to the usual laboratory test duration limited to a few thousands hours which is considered to be important from a life assessment point of view when assessing a manufacturing or in service flaw in a P91 component. It is crucial to avoid extrapolating the assessment results to an unpredicted value of C^* smaller than 10^-6 MJ/m²h (which relates to the normal service life of the component) using the available CCG parameters in this review.

As the most structural components are used under multiaxial stress condition, it is important to evaluate this effect when using CCG data from laboratory test to apply on actual structural component. Using Circumferentially Cracked Bar (CCB) specimen is a simple method to investigate multiaxial stress effect.^[21] Also, using CCB specimen does not need complicated test facilities and could be conducted in tubular furnaces. The authors are not aware of any test has been conducted on P91 material using CCB specimen. This could be an area of concern in any new research plan scheme.

Effect of service exposure time on P91

Mostly CCG data are obtained from new materials. However, in many cases, CCG assessment is needed for components which have already experienced certain degrees of creep damage. There are only few published data available on investigation of CCG behaviour of service exposed material. In an experiment,^[22] CCG test was conducted on P22 material after 110,000 hrs service exposure. The result indicated higher crack propagation rate by a factor of 3.1 compare to data obtained from new material. Similar study conducted within HIDA project on P22 and P91 materials^[20] which indicates little influence of service exposure. However the authors were not able to clarify the previous service condition of the specimens used in the study.

In general the concern about the high temperature/pressure service exposed materials is the creep cavity formation which may affect creep ductility and, consequently, CCG behaviour. However, it has been illustrated^[23] that grade P91 steel will undertake significant microstructure evolution after long-term service exposure by coarsening of existing M₂₃C₆ carbides, as well as intensive precipitation of coarse Laves phases. This process significantly affects the HAZ material by lower its creep strength which in many cases has resulted to pre-mature creep cracking known as Type IV crack.^[24] This in turn, implies that the extrapolation of short-term laboratory data can lead to an overestimation of the creep strength after long-term high temperature service exposure.^[25] This would indicates the requirement of having reliable CCG data from long-term laboratory testing to simulate the actual service condition or short term-laboratory testing on service exposed material from P91 steel.

Conclusion

Based on a review of the CCG data for P91 material, the analysis of the main parameters influencing CCG rates and the CCG life prediction using the existing models, the following conclusions were obtained:

1. The available CCG data for P91 parent and weld material do not fully satisfy the needs for an accurate component life assessment. The data exhibit a large scatter; a difference more than 20 in CCG rate was observed between the upper and lower bands in the low C^* regime. This in turn creates uncertainty in the level of conservatisms during the assessment. The present data suggest that there is little difference for cracking in P91 parent and weld material. Further works are necessary to precisely quantify and model the effect of different variables on P91 CCG data scatter band in order to quantify the difference between based and weld material. For the present to avoid either over-conservatism or under-estimation, it is strongly recommended, wherever is possible, the actual material data is obtained by laboratory testing and used during an assessment.
2. The available NSW model over conservatively bounds the data. By assuming that the damage angle at the crack tip controls the development of cracking, the NSW-MOD improves the analysis by reducing the upper-bound plane strain conservative prediction however the model still predicts an appropriate conservative upper limit for P91 CCG data. Further detailed information on material pedigree and creep properties is likely to improve the predictions using the NSW-MOD model.
3. There is no standard or procedure to help the users of life assessment codes to select the appropriate data relevant to their particular case from the data scatter band. Although it is crucial to have a wide range of data to be able to quantify the effect of different variable, however without a precise data selection procedure, there will be always a chance for underestimation or over-conservatism.
4. Apart from one study using Single Edge Notch Tension (SENT) specimen, no published data was found for P91 CCG using any other type of specimen. Other type of specimen should be explored to investigate the effect of constraint on parent, weld and HAZ P91 steel.
5. Long-term CCG data are not available for P91 steel. The implications in, constraint effects on CCG and metallurgical evolution of P91 after long-term service exposure, particularly at HAZ area, demand availability of long-term CCG data. This requirement could be satisfied by conducting long and short term experiments on ex-service exposed materials which have undertaken different stage of creep damage.

References

1. Ferritic pipe for high temperature use in boilers and petrochemical applications, The P91 book. France. Vallourec Industries : 1992.
2. Viswanathan R. Damage mechanisms and life assessment of high-temperature components. Ohio. ASTM Internationsl: 1990.
3. R5, An assessment procedure for the high temperature response of structures. Issue 3: British Energy 2003.
4. API/ASME 579: Fitness for Service, API/ASME 2007.
5. BS 7910: Guide to methods for assessing the acceptability of flaws in metallic structures, British Standard 2005.
6. RCC-MR Section 1, Sub-section 2, Technical Appendix A3, of RCC-MR; Design and construction rules for mechanical components of FBR nuclear islands. Afcen, Paris, 1985.
7. Webster G.A, Ainsworh R.A. High temperature component life assessment. London. Chapman & Hall: 1994.
8. ASTM, E1457-07e2: Measurement of creep crack growth times in metals, ASTM International 2007.
9. Tan, M., Celard, N.J.C., Nikbin, K.M. and Webster, G.A. 'Comparison of creep crack initiation and growth in four steels tested in HIDA', Int. J. Pres. Vessel and Piping. 2001; 78, 737-747.
10. Nikbin KM, Smith DJ, Webster G.A. An engineering approach to the prediction of creep crack growth. J. Eng. Mat. And Tech. ASME 108, pp186-191, 1986.
11. Yatomi M, O'Dowd N.P, Nikbin KM, Webster GA. Theoretical and numerical modelling of creep crack growth in a carbon-manganes steel. In: Journal of engineering fracture mechanics 73, 2006. p. 1158-75.
12. Nikbin K. Virtual testing and predictive modelling. Predicting Creep and Creep/Fatigue Crack Initiation and Growth for Virtual Testing and Life Assessment of Components, (Ed. B. Farahmand), Springer, 2009.
13. Wasmer K. Nikbin KM. Webster GA. A sensitivity study of creep crack growth in pipes. ASME PVP. High temp. of structures and materials. Vol. 438, pp:17-24.
14. Nikbin KM. Creep and creep/fatigue crack growth modelling and testing methods relevant to life assessment of cracked component. Welding Research Council Bulletin 512: 2006.
15. Davies CM, Wimpory RC, Tabuchi M, Dean DW, Nikbin K.M. The influence of specimen geometry and test times on high temperature crack growth behaviour in parent and welded 316H stainless steel. 2008 ASME Pressure Vessels and Piping Division Conference.
16. Nikbin KM. Comparison between crack growth in fracture mechanics specimens and feature component tests carried out in low alloys steel. Journal of pressure vessel technology, Vol.122, No.1, pp.40-45 (2000)
17. Wasmer K. Prediction of creep crack growth in a range of steel. A thesis submitted for the degree of doctor of philosophy of University of London and Diploma of Imperial College. May 2003.
18. Hamata N. Le Mat. Shibli I.A. creep crack growth of seam welded P22 and P91 pipes with artificial defcts. Par II. Data analysis. Int J Pres Ves Pip;78(2001):827-35
19. Al Abed B, HIDA project task II. ERA report 97-0586. July 1997
20. N. J. Celard, K. M. Nikbin, G. A. Webster. Validation, Expansion and Standardisation of Procedures for High Temperature Defect Assessment (HIDA). Final report of Imperial College London, April 2000.
21. Tabuchi et al. Creep crack growth properties for 12CrWCoB rotor steel using circular notched specimen. Int J Pres Ves Pip. 81(2004) 401-407.
22. Anderson CM, Sandstorm R. Creep crack growth in service exposed weld metal of 2.25Cr1Mo. Int J Pres Ves Pip. 78 (2001) 749-755.
23. Panait C, Bendick W, Fuchsmann A, Gourgues-Lorenzon A-F, Besson J. Study of the microstructure of the grade P91 steel after more than 100,000h of creep exposure at 600°C. Paper submitted to ECCC conference, April 2009.
24. Abson DJ, Rothwell JS. Review of Type IV Cracking in 9-12%Cr Creep-Resistant Martensitic Steels. TWI, Granta Park, Great Abington, Cambridgeshire, CB21 6AL, U.K.
25. Dimmler G, Weinert P, Cerjak H. Extrapolation of short-term creep rupture data - The potential risk of over-estimation. International Journal of Pressure Vessels and Piping 85 (2008) 55-62.